Lattice Boltzmann Numerics and Method Developement

The lattice Boltzmann method, ever since emergency in the early 90s has been known as an efficient alternative to classical numerical methods for the Navier-Stokes equations.

While coming at relatively low computational cost and very local discrete operators, owing -for the most part, to the discretization strategy of the particles speed space it has been plagued with stability issues. Stability issues have been serious limitations preventing extension to, among others, high Reynolds number and compressible flows.

The group has been a pioneer in the development of alternatives to the classical lattice Boltzmann formulation with extended domains of stability.

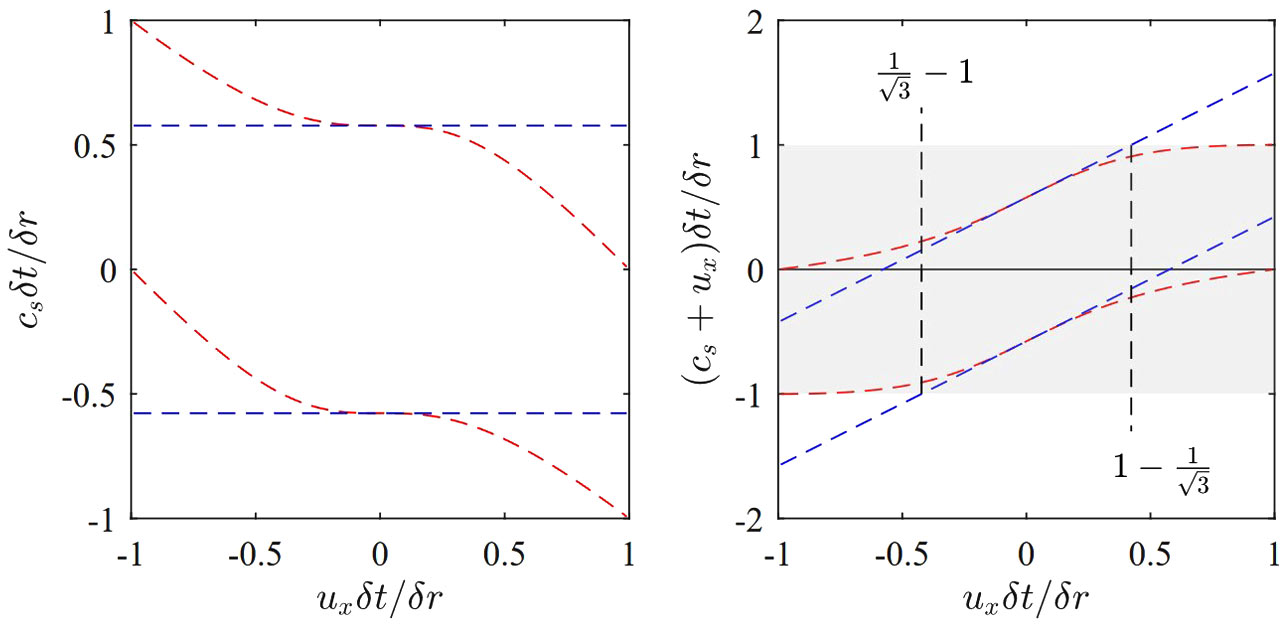
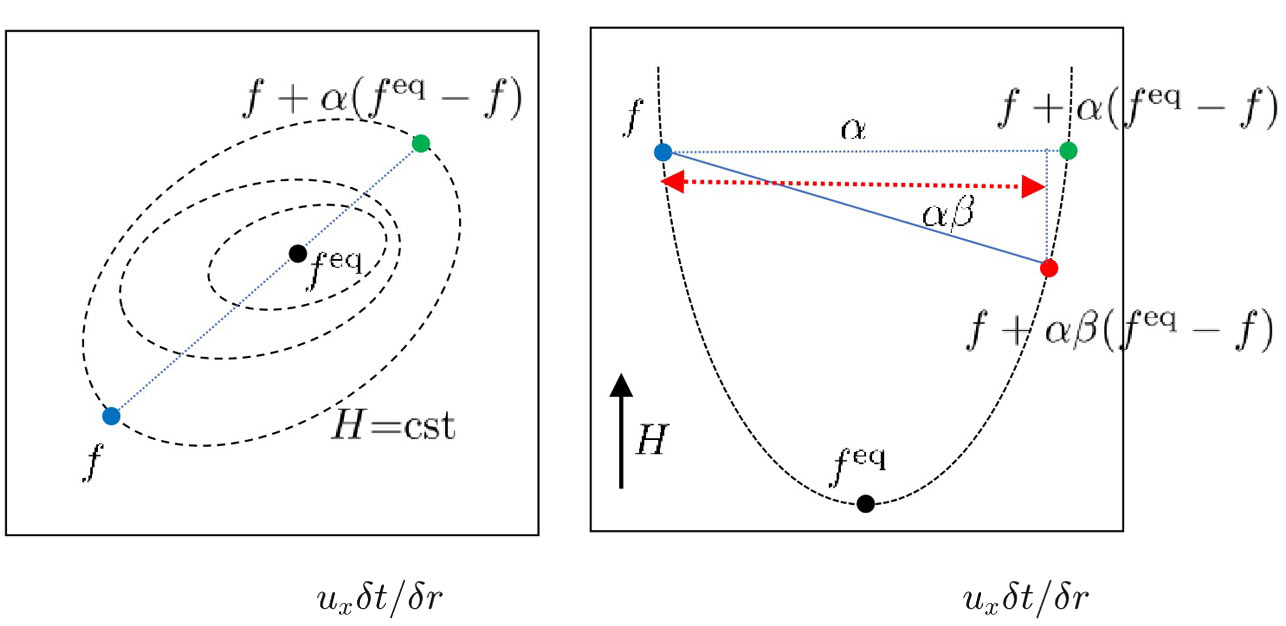
The entropic lattice Boltzmann method is one of these models with unconditional stability. We recently demonstrated that by allowing a certain degree of freedom in the equilibrium pressure the entropic construction of the discrete equilibrium state guarantees -contrary to polynomials alternatives strictly enforcing the diagonal second-order equilibrium moments- unconditional linear stability.

Enlarged view: (Left) Sound speed for entropic and polynomial equilibria as a function of velocity $u_x$. (Right) Comparison of the speed of fastest propagating eigen-modes: (blue dashed line) polynomial and (red line) entropic equilibria. — (Left) Sound speed for entropic and polynomial equilibria as a function of velocity $u_x$. (Right) Comparison of the speed of fastest propagating eigen-modes: (blue dashed line) polynomial and (red line) entropic equilibria.

In addition, the entropy-dictated relaxation process brings in a mechanism ensuring non-linear stability of the scheme.

Enlarged view: Illustration of entropic relaxation process. — Illustration of entropic relaxation process.

Enlarged view: Illustration of applications of the entropic multiple relaxation time model: (left) Flow over the SD7003 at ${\rm Re}=6\times10^{4}$ and (right) Cold flow in valve-piston assembly. — Illustration of applications of the entropic multiple relaxation time model: (left) Flow over the SD7003 at ${\rm Re}=6\times10^{4}$ and (right) Cold flow in valve-piston assembly.

Extensions of the entropic method to larger discrete lattices and compressible flows have been proposed.

Enlarged view: Drag coefficient $c_d$ as a function of the free stream Mach number for the Busemann biplane simulations. Inset: snapshots of the pressure distribution around the biplane for three different Mach numbers: Ma = 1.5, top; Ma = 1.7, bottom left; Ma = 2.0, bottom right. — Drag coefficient $c_d$ as a function of the free stream Mach number for the Busemann biplane simulations. Inset: snapshots of the pressure distribution around the biplane for three different Mach numbers: Ma = 1.5, top; Ma = 1.7, bottom left; Ma = 2.0, bottom right.

Related Publications

Entropic lattice Boltzmann models for hydrodynamics in three dimensions
Chikatamarla SS, Ansumali S, Karlin IV.
Physical review letters. 2006 Jul 7;97(1):010201.
Research Collection | external page https://doi.org/10.1103/PhysRevLett.97.010201

Entropic lattice Boltzmann methods: A review
Hosseini SA, Atif M, Ansumali S, Karlin IV.
Computers & Fluids. 2023 Mar 30:105884.
Research Collection | external page https://doi.org/10.3929/ethz-b-000608929

Entropic equilibrium for the lattice Boltzmann method: Hydrodynamics and numerical properties
Hosseini SA, Karlin IV.
arXiv preprint arXiv:2303.08163. 2023 Mar 14.
external page arXiv | https://doi.org/10.48550/arXiv.2303.08163