Kinetic Theory of Gases
Research is focused around Hilbert's sixth problem and the limit from kinetic theory to the macroscopic description of continua.
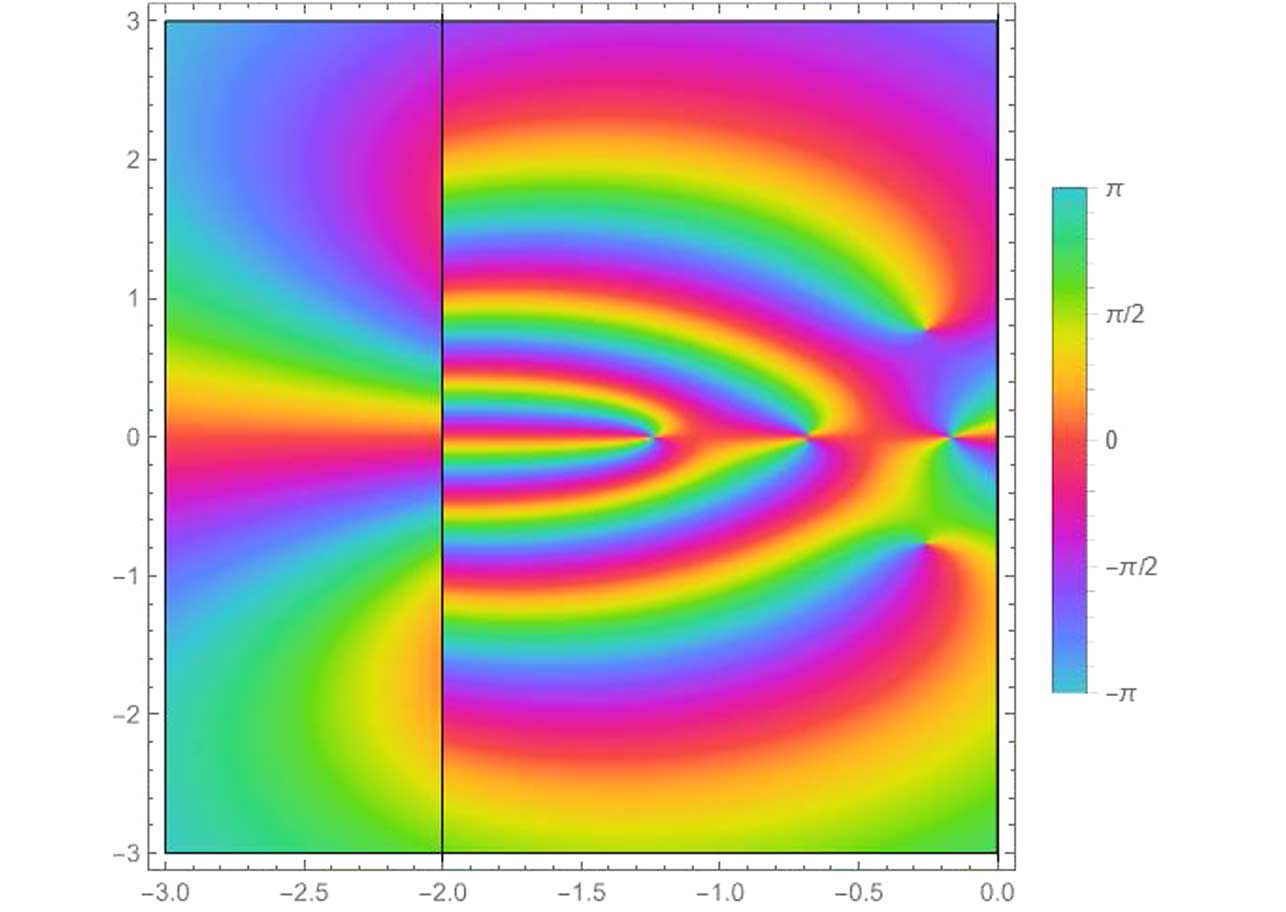
This line of research has a long a fruitful history - both in the context of applied mathematics as well for the analysis of PDEs. While the scaling limit for small relaxation times has been established, a complete description of the relation of collision processes on the microscopic and mean-field quantities in the macroscopic picture is still out of reach. Recent advances in the spectral theory of kinetic models (see Image) allow us to venture an attempt for a complete solution, especially in the context of consistent fluid models beyond Navier—Stokes.
Related Publications
Exact Hydrodynamic Manifolds for the Linearized Three-Dimensional Boltzmann BGK Equation
Kogelbauer F, Karlin I. E
arXiv preprint arXiv:2301.03069. 2023 Jan 8.
external page Research Gate | external page DOI:10.48550/arXiv.2301.03069
Spectral Analysis and Hydrodynamic Manifolds for the Linearized Shakhov Model
Kogelbauer F, Karlin I.
arXiv preprint arXiv:2305.06612. 2023 May 11.
external page Research Gate | external page DOI:10.2139/ssrn.4474785