High-Speed Compressible Flows
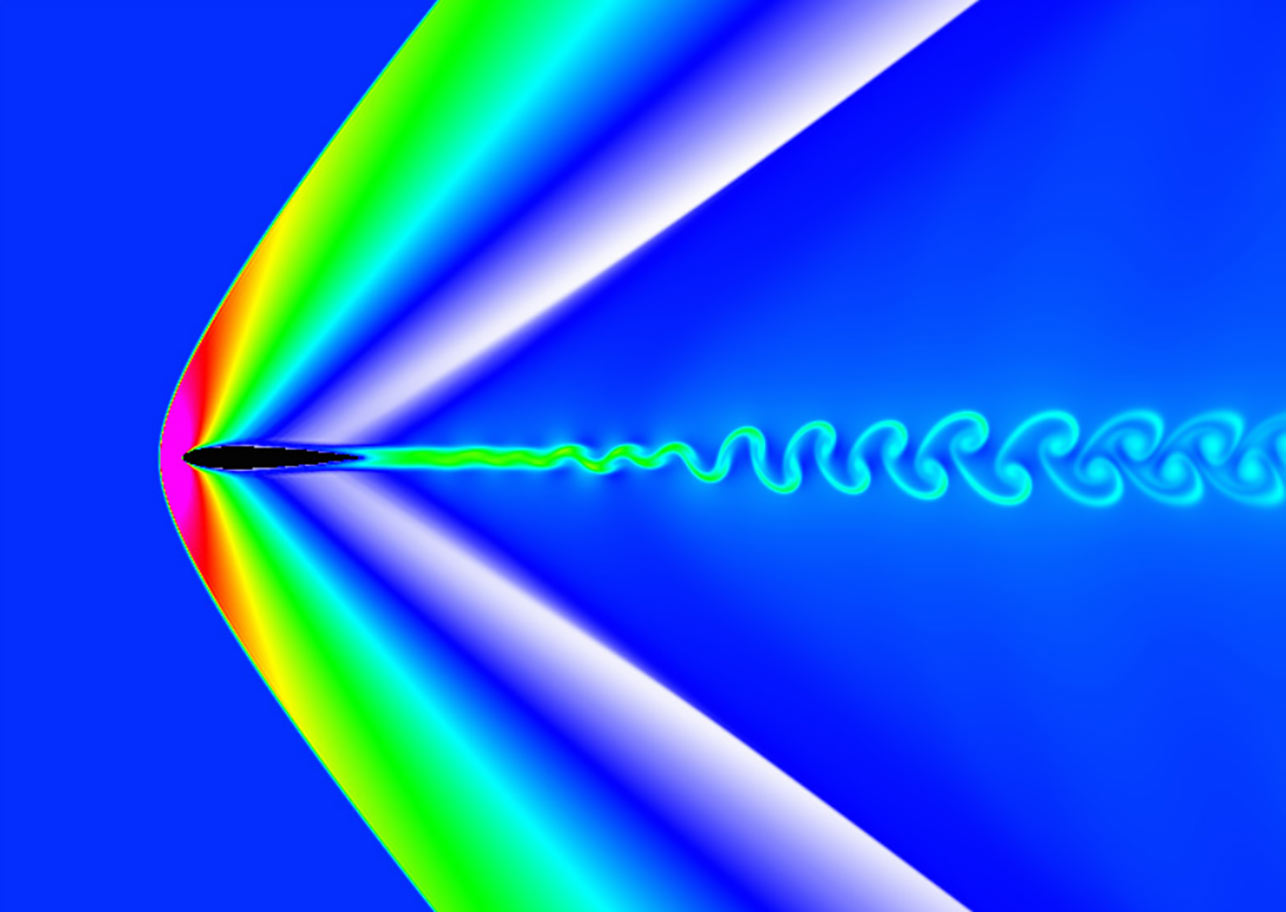
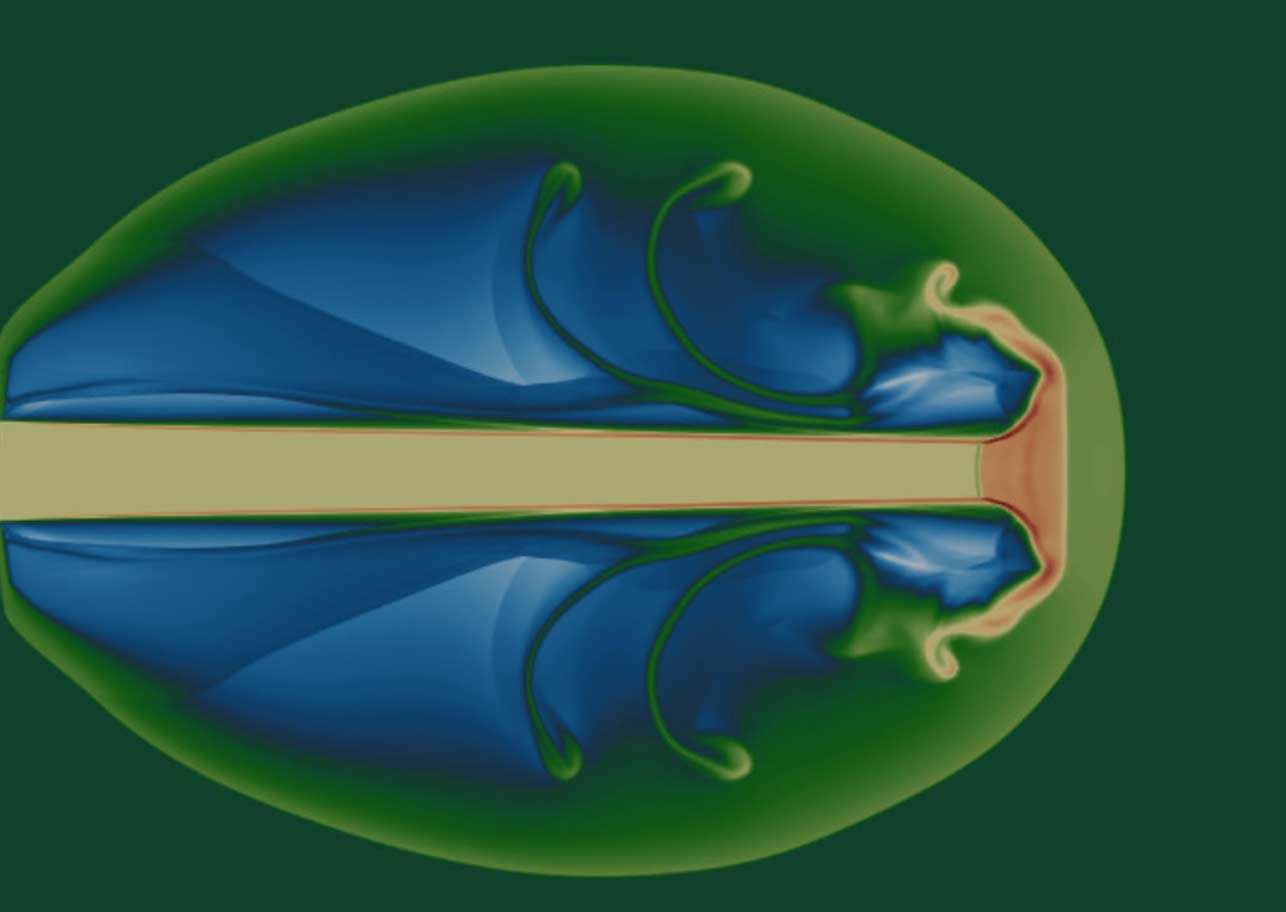
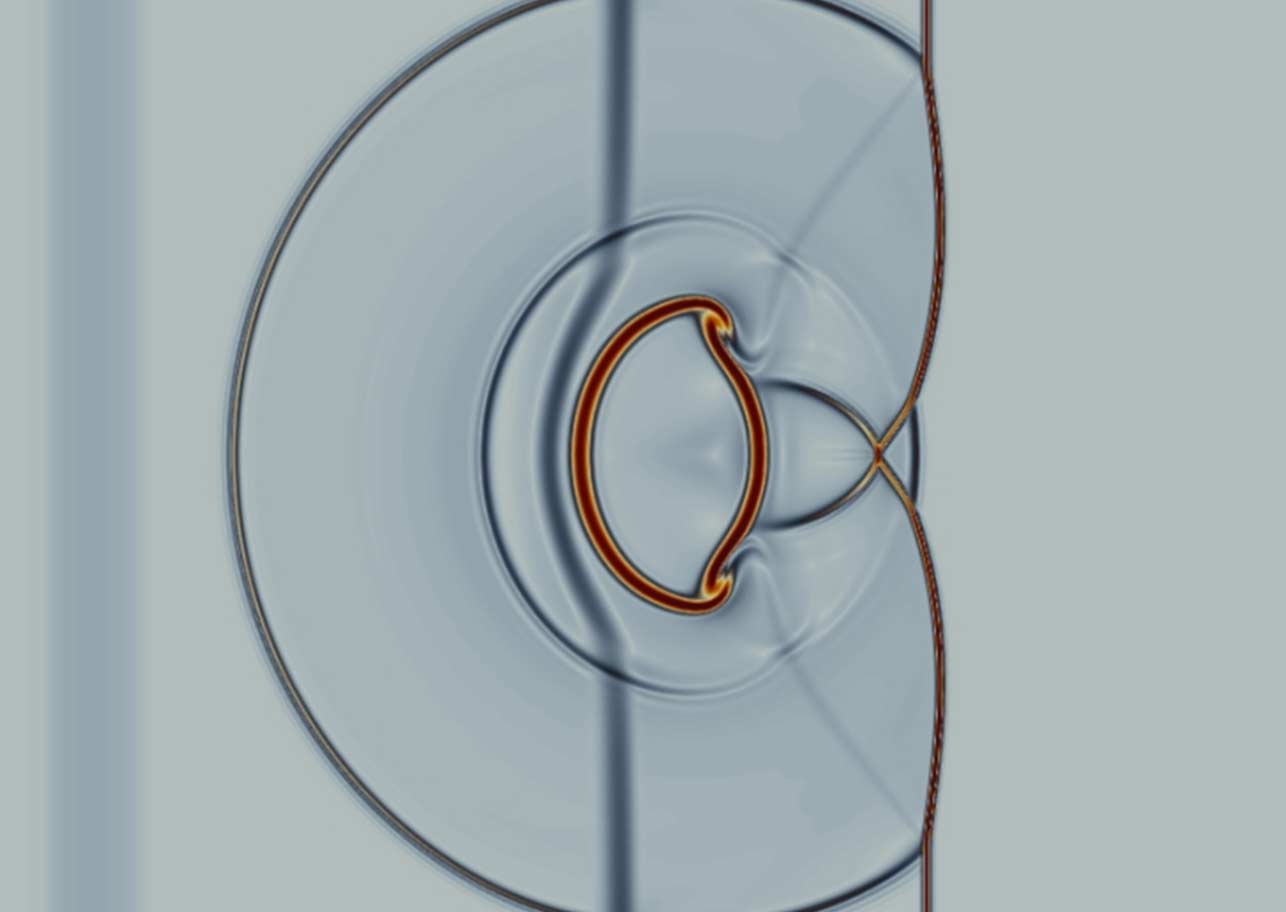
Accurate prediction of complex hydrodynamic features in high-speed compressible flows, including the propagation of shock waves and their interaction with boundaries is one of topics of interest in our group.
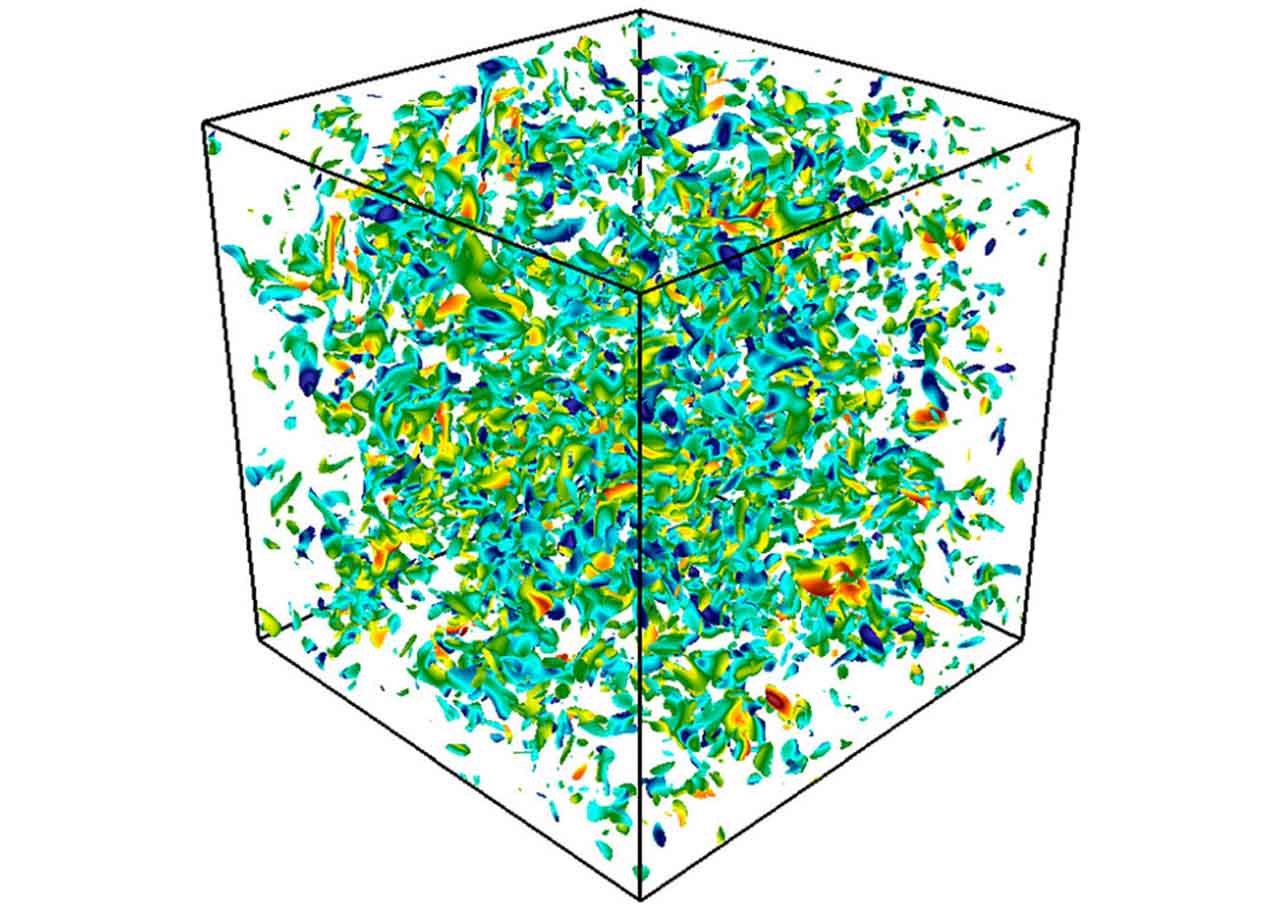
Despite the existence of numerous numerical algorithms, the high-speed compressible regime remains a challenging and active area of research within the scientific community.
Our group has been at the forefront of pioneering and actively developing two approaches in this field. The first approach focuses on a family of lattice Boltzmann models that preserve the on-lattice streaming, ensuring an efficient and numerically attractive implementation. By including additional populations, carefully designing equilibria, and employing stabilization techniques based on entropy arguments, these models enable the simulation of supersonic flows.
To push the boundaries even further and tackle higher speed and hypersonic flows, we have embarked on a drastic methodology change. Recently, we have successfully reformulated kinetic schemes with adaptive velocity sets, leading to an unprecedented range of achievable Mach numbers and temperature ratios. This innovative approach, known as the Particles on Demand (PonD) method, has undergone rigorous testing across various applications, including multiphase flows, combustion processes, and rarefied regimes, yielding promising results.
Recent developments based on the finite-volume method and application of an adaptive mesh refinement methodology show promising results for a strictly conservative and efficient simulation of compressible and high-speed flows.
Related Publications
Extended lattice Boltzmann model for gas dynamics
Saadat MH, Hosseini SA, Dorschner B, Karlin IV.
Physics of Fluids. 2021 Apr 14;33(4):046104.
Research Collection | external page https://doi.org/10.1063/5.0048029
Particles on demand for kinetic theory
Dorschner B, Bösch F, Karlin IV.
Physical review letters. 2018 Sep 25;121(13):130602.
Research Collection | external page https://doi.org/10.1103/PhysRevLett.121.130602
Particles on demand for flows with strong discontinuities
Kallikounis NG, Dorschner B, Karlin IV.
Physical Review E. 2022 Jul 5;106(1):015301.
Research Collection | external page https://doi.org/10.1103/PhysRevE.106.015301
A fully conservative discrete velocity Boltzmann solver with parallel adaptive mesh refinement for compressible flows
Strässle RM, Hosseini SA, Karlin IV.
Physics of Fluids 1 April 2025; 37(4):046110.
external page Research Collection | external page https://doi.org/10.1063/5.0263958